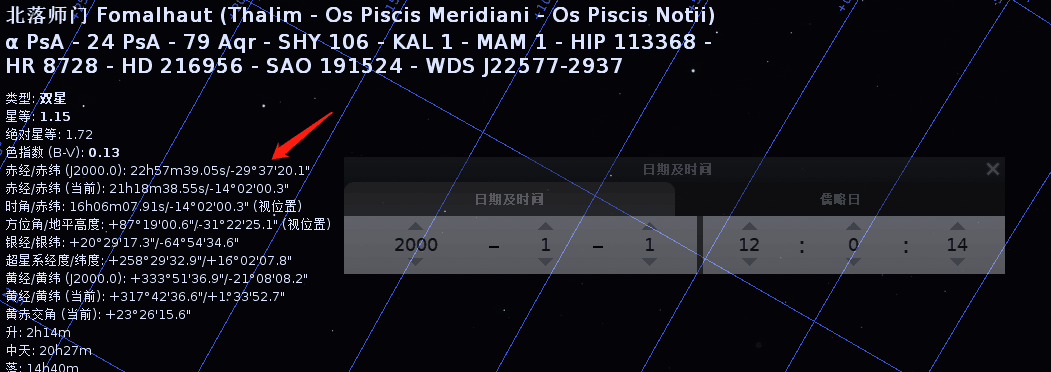
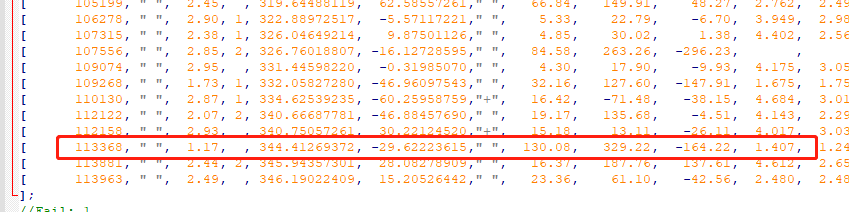
#include <bits/stdc++.h>
using namespace std;
#define BARYC 0
#define HELIOC 1
const double T0 = 2451545.00000000;
const double KMAU = 1.49597870e+8;
const double MAU = 1.49597870e+11;
const double C = 173.14463348;
const double GS = 1.32712438e+20;
const double TWOPI = 6.28318530717958647692;
const double RAD2SEC = 206264.806247096355;
const double DEG2RAD = 0.017453292519943296;
const double RAD2DEG = 57.295779513082321;
const double epoch_hip = 2448349.0625;
const double epoch_fk5 = 2451545.0000;
static double PSI_COR = 0.0;
static double EPS_COR = 0.0;
const short int FN0 = 0;
typedef struct
{
long int starnumber;
double ra;
double dec;
double promora;
double promodec;
double parallax;
double radialvelocity;
} cat_entry;
typedef struct
{
double latitude;
double longitude;
} site_info;
short int vector2radec(double *pos, double *ra, double *dec)
{
double xyproj;
xyproj = sqrt(pow(pos[0], 2.0) + pow(pos[1], 2.0));
if ((xyproj == 0.0) && (pos[2] == 0))
{
*ra = 0.0;
*dec = 0.0;
return 1;
}
else if (xyproj == 0.0)
{
*ra = 0.0;
if (pos[2] < 0.0)
*dec = -90.0;
else
*dec = 90.0;
return 2;
}
else
{
*ra = atan2(pos[1], pos[0]) * RAD2SEC / 54000.0;
*dec = atan2(pos[2], xyproj) * RAD2SEC / 3600.0;
if (*ra < 0.0)
*ra += 24.0;
}
return 0;
}
void fund_args(double t, double a[5])
{
short int i;
a[0] = 2.3555483935439407 + t * (8328.691422883896 + t * (1.517951635553957e-4 + 3.1028075591010306e-7 * t));
a[1] = 6.240035939326023 + t * (628.3019560241842 + t * (-2.7973749400020225e-6 - 5.817764173314431e-8 * t));
a[2] = 1.6279019339719611 + t * (8433.466158318453 + t * (-6.427174970469119e-5 + 5.332950492204896e-8 * t));
a[3] = 5.198469513579922 + t * (7771.377146170642 + t * (-3.340851076525812e-5 + 9.211459941081184e-8 * t));
a[4] = 2.1824386243609943 + t * (-33.75704593375351 + t * (3.614285992671591e-5 + 3.878509448876288e-8 * t));
for (i = 0; i < 5; i++)
{
a[i] = fmod(a[i], TWOPI);
if (a[i] < 0.0)
a[i] += TWOPI;
}
return;
}
short int nutation_angles(double t, double *longnutation, double *obliqnutation)
{
double clng[106] = {1.0, 1.0, -1.0, -1.0, 1.0, -1.0, -1.0,
-1.0, -1.0, -1.0, -1.0, 1.0, -1.0, 1.0,
-1.0, 1.0, 1.0, -1.0, -1.0, 1.0, 1.0,
-1.0, 1.0, -1.0, 1.0, -1.0, -1.0, -1.0,
1.0, -1.0, -1.0, 1.0, -1.0, 1.0, 2.0,
2.0, 2.0, 2.0, 2.0, -2.0, 2.0, 2.0,
2.0, 3.0, -3.0, -3.0, 3.0, -3.0, 3.0,
-3.0, 3.0, 4.0, 4.0, -4.0, -4.0, 4.0,
-4.0, 5.0, 5.0, 5.0, -5.0, 6.0, 6.0,
6.0, -6.0, 6.0, -7.0, 7.0, 7.0, -7.0,
-8.0, 10.0, 11.0, 12.0, -13.0, -15.0, -16.0,
-16.0, 17.0, -21.0, -22.0, 26.0, 29.0, 29.0,
-31.0, -38.0, -46.0, 48.0, -51.0, 58.0, 59.0,
63.0, 63.0, -123.0, 129.0, -158.0, -217.0, -301.0,
-386.0, -517.0, 712.0, 1426.0, 2062.0, -2274.0,
-13187.0, -171996.0},
clngx[14] = {0.1, -0.1, 0.1, 0.1, 0.1, 0.1, 0.2, -0.2, -0.4, 0.5, 1.2,
-1.6, -3.4, -174.2},
cobl[64] = {1.0, 1.0, 1.0, -1.0, -1.0, -1.0,
1.0, 1.0, 1.0, 1.0, 1.0, -1.0,
1.0, -1.0, 1.0, -1.0, -1.0, -1.0,
1.0, -1.0, 1.0, 1.0, -1.0, -2.0,
-2.0, -2.0, 3.0, 3.0, -3.0, 3.0,
3.0, -3.0, 3.0, 3.0, -3.0, 3.0,
3.0, 5.0, 6.0, 7.0, -7.0, 7.0,
-8.0, 9.0, -10.0, -12.0, 13.0, 16.0,
-24.0, 26.0, 27.0, 32.0, -33.0, -53.0,
54.0, -70.0, -95.0, 129.0, 200.0, 224.0,
-895.0, 977.0, 5736.0, 92025.0},
coblx[8] = {-0.1, -0.1, 0.3, 0.5, -0.5, -0.6, -3.1, 8.9};
short int i, ii, i1, i2, iop;
short int nav1[10] = {0, 0, 1, 0, 2, 1, 3, 0, 4, 0},
nav2[10] = {0, 0, 0, 5, 1, 1, 3, 3, 4, 4},
nav[183] = {2, 0, 1, 1, 5, 2, 2, 0, 2, 1, 0, 3, 2, 5, 8, 1, 17, 8,
1, 18, 0, 2, 0, 8, 0, 1, 3, 2, 1, 8, 0, 17, 1, 1, 15, 1,
2, 21, 1, 1, 2, 8, 2, 0, 29, 1, 21, 2, 2, 1, 29, 2, 0, 9,
2, 5, 4, 2, 0, 4, 0, 1, 9, 2, 1, 4, 0, 2, 9, 2, 2, 4,
1, 14, 44, 2, 0, 45, 2, 5, 44, 2, 50, 0, 1, 36, 2, 2, 5, 45,
1, 37, 2, 2, 1, 45, 2, 1, 44, 2, 53, 1, 2, 8, 4, 1, 40, 3,
2, 17, 4, 2, 0, 64, 1, 39, 8, 2, 27, 4, 1, 50, 18, 1, 21, 47,
2, 44, 3, 2, 44, 8, 2, 45, 8, 1, 46, 8, 0, 67, 2, 1, 5, 74,
1, 0, 74, 2, 50, 8, 1, 5, 78, 2, 17, 53, 2, 53, 8, 2, 0, 80,
2, 0, 81, 0, 7, 79, 1, 7, 81, 2, 1, 81, 2, 24, 44, 1, 1, 79,
2, 27, 44},
llng[106] = {57, 25, 82, 34, 41, 66, 33, 36, 19, 88, 18, 104, 93,
84, 47, 28, 83, 86, 69, 75, 89, 30, 58, 73, 46, 77,
23, 32, 59, 72, 31, 16, 74, 22, 98, 38, 62, 96, 37,
35, 6, 76, 85, 51, 26, 10, 13, 63, 105, 52, 102, 67,
99, 15, 24, 14, 3, 100, 65, 11, 55, 68, 20, 87, 64,
95, 27, 60, 61, 80, 91, 94, 12, 43, 71, 42, 97, 70,
7, 49, 29, 2, 5, 92, 50, 78, 56, 17, 48, 40, 90,
8, 39, 54, 81, 21, 103, 53, 45, 101, 0, 1, 9, 44,
79, 4},
llngx[14] = {81, 7, 97, 0, 39, 40, 9, 44, 45, 103, 101, 79, 1, 4},
lobl[64] = {51, 98, 17, 21, 5, 2, 63, 105, 38, 52, 102, 62, 96,
37, 35, 76, 36, 88, 85, 104, 93, 84, 83, 67, 99, 8,
68, 100, 60, 61, 91, 87, 64, 80, 95, 65, 55, 94, 43,
97, 0, 71, 70, 42, 49, 92, 50, 78, 56, 90, 48, 40,
39, 54, 1, 81, 103, 53, 45, 101, 9, 44, 79, 4},
loblx[8] = {53, 1, 103, 9, 44, 101, 79, 4};
double a[5], angle, cc, ss1, cs, sc, c[106], s[106], lng, lngx, obl,
oblx;
fund_args(t, a);
i = 0;
for (ii = 0; ii < 10; ii += 2)
{
angle = a[nav1[ii]] * (double)(nav1[1 + ii] + 1);
c[i] = cos(angle);
s[i] = sin(angle);
i += 1;
}
i = 5;
for (ii = 0; ii < 10; ii += 2)
{
i1 = nav2[ii];
i2 = nav2[1 + ii];
c[i] = c[i1] * c[i2] - s[i1] * s[i2];
s[i] = s[i1] * c[i2] + c[i1] * s[i2];
i += 1;
}
i = 10;
for (ii = 0; ii < 183; ii += 3)
{
iop = nav[ii];
i1 = nav[1 + ii];
i2 = nav[2 + ii];
switch (iop)
{
case 0:
c[i] = c[i1] * c[i2] - s[i1] * s[i2];
s[i] = s[i1] * c[i2] + c[i1] * s[i2];
i += 1;
break;
case 1:
c[i] = c[i1] * c[i2] + s[i1] * s[i2];
s[i] = s[i1] * c[i2] - c[i1] * s[i2];
i += 1;
break;
case 2:
cc = c[i1] * c[i2];
ss1 = s[i1] * s[i2];
sc = s[i1] * c[i2];
cs = c[i1] * s[i2];
c[i] = cc - ss1;
s[i] = sc + cs;
i += 1;
c[i] = cc + ss1;
s[i] = sc - cs;
i += 1;
break;
}
if (iop == 3)
break;
}
lng = 0.0;
for (i = 0; i < 106; i++)
lng += clng[i] * s[llng[i]];
lngx = 0.0;
for (i = 0; i < 14; i++)
lngx += clngx[i] * s[llngx[i]];
obl = 0.0;
for (i = 0; i < 64; i++)
obl += cobl[i] * c[lobl[i]];
oblx = 0.0;
for (i = 0; i < 8; i++)
oblx += coblx[i] * c[loblx[i]];
*longnutation = (lng + t * lngx) / 10000.0;
*obliqnutation = (obl + t * oblx) / 10000.0;
return 0;
}
void earthtilt(double tjd, double *mobl, double *tobl, double *eq, double *dpsi, double *deps)
{
static double tjd_last = 0.0;
static double t, dp, de;
double mean_obliq, true_obliq, eq_eq, args[5];
t = (tjd - T0) / 36525.0;
if (fabs(tjd - tjd_last) > 1.0e-6)
nutation_angles(t, &dp, &de);
mean_obliq = 84381.4480 - 46.8150 * t - 0.00059 * pow(t, 2.0) + 0.001813 * pow(t, 3.0);
true_obliq = mean_obliq + de;
mean_obliq /= 3600.0;
true_obliq /= 3600.0;
fund_args(t, args);
eq_eq = dp * cos(mean_obliq * DEG2RAD) +
(0.00264 * sin(args[4]) + 0.000063 * sin(2.0 * args[4]));
eq_eq /= 15.0;
*dpsi = dp;
*deps = de;
*eq = eq_eq;
*mobl = mean_obliq;
*tobl = true_obliq;
return;
}
void starvectors(cat_entry *star, double *pos, double *vel)
{
double paralx, dist, r, d, cra, sra, cdc, sdc, pmr, pmd, rvl;
paralx = star->parallax;
if (star->parallax <= 0.0)
paralx = 1.0e-7;
dist = RAD2SEC / paralx;
r = (star->ra) * 15.0 * DEG2RAD;
d = (star->dec) * DEG2RAD;
cra = cos(r);
sra = sin(r);
cdc = cos(d);
sdc = sin(d);
pos[0] = dist * cdc * cra;
pos[1] = dist * cdc * sra;
pos[2] = dist * sdc;
pmr = star->promora * 15.0 * cdc / (paralx * 36525.0);
pmd = star->promodec / (paralx * 36525.0);
rvl = star->radialvelocity * 86400.0 / KMAU;
vel[0] = -pmr * sra - pmd * sdc * cra + rvl * cdc * cra;
vel[1] = pmr * cra - pmd * sdc * sra + rvl * cdc * sra;
vel[2] = pmd * cdc + rvl * sdc;
return;
}
void tdb2tdt(double tdb, double *tdtjd, double *secdiff)
{
double ecc = 0.01671022;
double rev = 1296000.0;
double tdays, m, l, lj, e;
tdays = tdb - T0;
m = (357.51716 + 0.985599987 * tdays) * 3600.0;
l = (280.46435 + 0.985609100 * tdays) * 3600.0;
lj = (34.40438 + 0.083086762 * tdays) * 3600.0;
m = fmod(m, rev) / RAD2SEC;
l = fmod(l, rev) / RAD2SEC;
lj = fmod(lj, rev) / RAD2SEC;
e = m + ecc * sin(m) + 0.5 * ecc * ecc * sin(2.0 * m);
*secdiff = 1.658e-3 * sin(e) + 20.73e-6 * sin(l - lj);
*tdtjd = tdb - *secdiff / 86400.0;
return;
}
void precession(double tjd1, double *pos, double tjd2, double *pos2);
void transform_cat(cat_entry *incat, cat_entry *newcat)
{
short int j;
double paralx, dist, r, d, cra, sra, cdc, sdc, pos1[3], term1, pmr, pmd, rvl, vel1[3], pos2[3], vel2[3], xyproj;
paralx = incat->parallax;
if (paralx <= 0.0)
paralx = 1.0e-7;
dist = RAD2SEC / paralx;
r = incat->ra * 54000.0 / RAD2SEC;
d = incat->dec * 3600.0 / RAD2SEC;
cra = cos(r);
sra = sin(r);
cdc = cos(d);
sdc = sin(d);
pos1[0] = dist * cdc * cra;
pos1[1] = dist * cdc * sra;
pos1[2] = dist * sdc;
term1 = paralx * 36525.0;
pmr = incat->promora * 15.0 * cdc / term1;
pmd = incat->promodec / term1;
vel1[0] = -pmr * sra - pmd * sdc * cra;
vel1[1] = pmr * cra - pmd * sdc * sra;
vel1[2] = pmd * cdc;
for (j = 0; j < 3; j++)
{
pos2[j] = pos1[j] + vel1[j] * (epoch_fk5 - epoch_hip);
vel2[j] = vel1[j];
}
xyproj = sqrt(pos2[0] * pos2[0] + pos2[1] * pos2[1]);
r = atan2(pos2[1], pos2[0]);
d = atan2(pos2[2], xyproj);
newcat->ra = r * RAD2SEC / 54000.0;
newcat->dec = d * RAD2SEC / 3600.0;
if (newcat->ra < 0.0)
newcat->ra += 24.0;
dist = sqrt(pos2[0] * pos2[0] + pos2[1] * pos2[1] +
pos2[2] * pos2[2]);
paralx = RAD2SEC / dist;
newcat->parallax = paralx;
cra = cos(r);
sra = sin(r);
cdc = cos(d);
sdc = sin(d);
pmr = -vel2[0] * sra + vel2[1] * cra;
pmd = -vel2[0] * cra * sdc - vel2[1] * sra * sdc + vel2[2] * cdc;
rvl = vel2[0] * cra * cdc + vel2[1] * sra * cdc + vel2[2] * sdc;
newcat->promora = pmr * paralx * 36525.0 / (15.0 * cdc);
newcat->promodec = pmd * paralx * 36525.0;
newcat->radialvelocity = rvl * KMAU / 86400.0;
if (newcat->parallax <= 1.01e-7)
{
newcat->parallax = 0.0;
newcat->radialvelocity = incat->radialvelocity;
}
newcat->starnumber = incat->starnumber;
return;
}
void transform_hip(cat_entry *hipparcos, cat_entry *fk5)
{
cat_entry scratch;
scratch.starnumber = hipparcos->starnumber;
scratch.dec = hipparcos->dec;
scratch.radialvelocity = hipparcos->radialvelocity;
scratch.ra = hipparcos->ra / 15.0;
scratch.promora = hipparcos->promora / (150.0 *
cos(hipparcos->dec * DEG2RAD));
scratch.promodec = hipparcos->promodec / 10.0;
scratch.parallax = hipparcos->parallax / 1000.0;
transform_cat(&scratch, fk5);
return;
}
void sun_eph(double jd, double *ra, double *dec, double *dis)
{
short int i;
double sum_lon = 0.0;
double sum_r = 0.0;
const double factor = 1.0e-07;
double u, arg, lon, lat, t, t2, emean, sin_lon;
struct sun_con
{
double l;
double r;
double alpha;
double nu;
};
static const struct sun_con con[50] =
{{403406.0, 0.0, 4.721964, 1.621043},
{195207.0, -97597.0, 5.937458, 62830.348067},
{119433.0, -59715.0, 1.115589, 62830.821524},
{112392.0, -56188.0, 5.781616, 62829.634302},
{3891.0, -1556.0, 5.5474, 125660.5691},
{2819.0, -1126.0, 1.5120, 125660.9845},
{1721.0, -861.0, 4.1897, 62832.4766},
{0.0, 941.0, 1.163, 0.813},
{660.0, -264.0, 5.415, 125659.310},
{350.0, -163.0, 4.315, 57533.850},
{334.0, 0.0, 4.553, -33.931},
{314.0, 309.0, 5.198, 777137.715},
{268.0, -158.0, 5.989, 78604.191},
{242.0, 0.0, 2.911, 5.412},
{234.0, -54.0, 1.423, 39302.098},
{158.0, 0.0, 0.061, -34.861},
{132.0, -93.0, 2.317, 115067.698},
{129.0, -20.0, 3.193, 15774.337},
{114.0, 0.0, 2.828, 5296.670},
{99.0, -47.0, 0.52, 58849.27},
{93.0, 0.0, 4.65, 5296.11},
{86.0, 0.0, 4.35, -3980.70},
{78.0, -33.0, 2.75, 52237.69},
{72.0, -32.0, 4.50, 55076.47},
{68.0, 0.0, 3.23, 261.08},
{64.0, -10.0, 1.22, 15773.85},
{46.0, -16.0, 0.14, 188491.03},
{38.0, 0.0, 3.44, -7756.55},
{37.0, 0.0, 4.37, 264.89},
{32.0, -24.0, 1.14, 117906.27},
{29.0, -13.0, 2.84, 55075.75},
{28.0, 0.0, 5.96, -7961.39},
{27.0, -9.0, 5.09, 188489.81},
{27.0, 0.0, 1.72, 2132.19},
{25.0, -17.0, 2.56, 109771.03},
{24.0, -11.0, 1.92, 54868.56},
{21.0, 0.0, 0.09, 25443.93},
{21.0, 31.0, 5.98, -55731.43},
{20.0, -10.0, 4.03, 60697.74},
{18.0, 0.0, 4.27, 2132.79},
{17.0, -12.0, 0.79, 109771.63},
{14.0, 0.0, 4.24, -7752.82},
{13.0, -5.0, 2.01, 188491.91},
{13.0, 0.0, 2.65, 207.81},
{13.0, 0.0, 4.98, 29424.63},
{12.0, 0.0, 0.93, -7.99},
{10.0, 0.0, 2.21, 46941.14},
{10.0, 0.0, 3.59, -68.29},
{10.0, 0.0, 1.50, 21463.25},
{10.0, -9.0, 2.55, 157208.40}};
u = (jd - T0) / 3652500.0;
for (i = 0; i < 50; i++)
{
arg = con[i].alpha + con[i].nu * u;
sum_lon += con[i].l * sin(arg);
sum_r += con[i].r * cos(arg);
}
lon = 4.9353929 + 62833.1961680 * u + factor * sum_lon;
lon = fmod(lon, TWOPI);
if (lon < 0.0)
lon += TWOPI;
lat = 0.0;
*dis = 1.0001026 + factor * sum_r;
t = u * 100.0;
t2 = t * t;
emean = (0.001813 * t2 * t - 0.00059 * t2 - 46.8150 * t + 84381.448) / RAD2SEC;
sin_lon = sin(lon);
*ra = atan2((cos(emean) * sin_lon), cos(lon)) * RAD2DEG;
*ra = fmod(*ra, 360.0);
if (*ra < 0.0)
*ra += 360.0;
*ra = *ra / 15.0;
*dec = asin(sin(emean) * sin_lon) * RAD2DEG;
return;
}
void precession(double tjd1, double *pos, double tjd2, double *pos2)
{
double xx, yx, zx, xy, yy, zy, xz, yz, zz, t, t1, t02, t2, t3, zeta0, zee, theta;
t = (tjd1 - T0) / 36525.0;
t1 = (tjd2 - tjd1) / 36525.0;
t02 = t * t;
t2 = t1 * t1;
t3 = t2 * t1;
zeta0 = (2306.2181 + 1.39656 * t - 0.000139 * t02) * t1 + (0.30188 - 0.000344 * t) * t2 + 0.017998 * t3;
zee = (2306.2181 + 1.39656 * t - 0.000139 * t02) * t1 + (1.09468 + 0.000066 * t) * t2 + 0.018203 * t3;
theta = (2004.3109 - 0.85330 * t - 0.000217 * t02) * t1 + (-0.42665 - 0.000217 * t) * t2 - 0.041833 * t3;
zeta0 /= RAD2SEC;
zee /= RAD2SEC;
theta /= RAD2SEC;
xx = cos(zeta0) * cos(theta) * cos(zee) - sin(zeta0) * sin(zee);
yx = -sin(zeta0) * cos(theta) * cos(zee) - cos(zeta0) *
sin(zee);
zx = -sin(theta) * cos(zee);
xy = cos(zeta0) * cos(theta) * sin(zee) + sin(zeta0) * cos(zee);
yy = -sin(zeta0) * cos(theta) * sin(zee) + cos(zeta0) *
cos(zee);
zy = -sin(theta) * sin(zee);
xz = cos(zeta0) * sin(theta);
yz = -sin(zeta0) * sin(theta);
zz = cos(theta);
pos2[0] = xx * pos[0] + yx * pos[1] + zx * pos[2];
pos2[1] = xy * pos[0] + yy * pos[1] + zy * pos[2];
pos2[2] = xz * pos[0] + yz * pos[1] + zz * pos[2];
return;
}
void radec2vector(double ra, double dec, double dist, double *vector)
{
vector[0] = dist * cos(DEG2RAD * dec) * cos(DEG2RAD * 15.0 * ra);
vector[1] = dist * cos(DEG2RAD * dec) * sin(DEG2RAD * 15.0 * ra);
vector[2] = dist * sin(DEG2RAD * dec);
return;
}
short int solarsystem(double tjd, short int origin, double *pos, double *vel)
{
short int ierr = 0;
short int i;
const double pm[4] = {1047.349, 3497.898, 22903.0, 19412.2};
const double pa[4] = {5.203363, 9.537070, 19.191264, 30.068963};
const double pl[4] = {0.600470, 0.871693, 5.466933, 5.321160};
const double pn[4] = {1.450138e-3, 5.841727e-4, 2.047497e-4, 1.043891e-4};
const double obl = 23.43929111;
static double tlast = 0.0;
static double sine, cose, tmass, pbary[3], vbary[3];
double oblr, qjd, ras, decs, diss, pos1[3], p[3][3], dlon, sinl, cosl, x, y, z, xdot, ydot, zdot, f;
if (tlast == 0.0)
{
oblr = obl * TWOPI / 360.0;
sine = sin(oblr);
cose = cos(oblr);
tmass = 1.0;
for (i = 0; i < 4; i++)
tmass += 1.0 / pm[i];
tlast = 1.0;
}
if ((tjd < 2340000.5) || (tjd > 2560000.5))
return (ierr = 1);
for (i = 0; i < 3; i++)
{
qjd = tjd + (double)(i - 1) * 0.1;
sun_eph(qjd, &ras, &decs, &diss);
radec2vector(ras, decs, diss, pos1);
precession(qjd, pos1, T0, pos);
p[i][0] = -pos[0];
p[i][1] = -pos[1];
p[i][2] = -pos[2];
}
for (i = 0; i < 3; i++)
{
pos[i] = p[1][i];
vel[i] = (p[2][i] - p[0][i]) / 0.2;
}
if (origin == 0)
{
if (fabs(tjd - tlast) >= 1.0e-06)
{
for (i = 0; i < 3; i++)
pbary[i] = vbary[i] = 0.0;
for (i = 0; i < 4; i++)
{
dlon = pl[i] + pn[i] * (tjd - T0);
dlon = fmod(dlon, TWOPI);
sinl = sin(dlon);
cosl = cos(dlon);
x = pa[i] * cosl;
y = pa[i] * sinl * cose;
z = pa[i] * sinl * sine;
xdot = -pa[i] * pn[i] * sinl;
ydot = pa[i] * pn[i] * cosl * cose;
zdot = pa[i] * pn[i] * cosl * sine;
f = 1.0 / (pm[i] * tmass);
pbary[0] += x * f;
pbary[1] += y * f;
pbary[2] += z * f;
vbary[0] += xdot * f;
vbary[1] += ydot * f;
vbary[2] += zdot * f;
}
tlast = tjd;
}
for (i = 0; i < 3; i++)
{
pos[i] -= pbary[i];
vel[i] -= vbary[i];
}
}
return (ierr);
}
void proper_motion(double tjd1, double *pos, double *vel, double tjd2, double *pos2)
{
short int j;
for (j = 0; j < 3; j++)
pos2[j] = pos[j] + (vel[j] * (tjd2 - tjd1));
return;
}
short int get_earth(double tjd, double *tdb, double *bary_earthp, double *bary_earthv, double *helio_earthp, double *helio_earthv)
{
short int error = 0;
static double tjd_last = 0.0;
static double time1, peb[3], veb[3], pes[3], ves[3];
double dummy, secdiff;
if (fabs(tjd - tjd_last) > 1.0e-6)
{
tdb2tdt(tjd, &dummy, &secdiff);
time1 = tjd + secdiff / 86400.0;
if (error = solarsystem(time1, BARYC, peb, veb))
{
tjd_last = 0.0;
return error;
}
if (error = solarsystem(time1, HELIOC, pes, ves))
{
tjd_last = 0.0;
return error;
}
tjd_last = tjd;
}
*tdb = time1;
for (short int i = 0; i < 3; i++)
{
bary_earthp[i] = peb[i];
bary_earthv[i] = veb[i];
helio_earthp[i] = pes[i];
helio_earthv[i] = ves[i];
}
return error;
}
void bary_to_geo(double *pos, double *earthvector, double *pos2, double *lighttime)
{
short int j;
double sum_of_squares;
for (j = 0; j < 3; j++)
pos2[j] = pos[j] - earthvector[j];
sum_of_squares = pow(pos2[0], 2.0) + pow(pos2[1], 2.0) + pow(pos2[2], 2.0);
*lighttime = sqrt(sum_of_squares) / C;
return;
}
short int sun_field(double *pos, double *earthvector, double *pos2)
{
short int j;
double f = 0.0;
double p1mag, pemag, cosd, sind, b, bm, pqmag, zfinl, zinit,
xifinl, xiinit, delphi, delphp, delp, p1hat[3], pehat[3];
double c = (C * MAU) / 86400.0;
p1mag = sqrt(pow(pos[0], 2.0) + pow(pos[1], 2.0) + pow(pos[2], 2.0));
pemag = sqrt(pow(earthvector[0], 2.0) + pow(earthvector[1], 2.0) + pow(earthvector[2], 2.0));
for (j = 0; j < 3; j++)
{
p1hat[j] = pos[j] / p1mag;
pehat[j] = earthvector[j] / pemag;
}
cosd = -pehat[0] * p1hat[0] - pehat[1] * p1hat[1] - pehat[2] * p1hat[2];
if (fabs(cosd) > 0.9999999999)
{
for (j = 0; j < 3; j++)
pos2[j] = pos[j];
}
else
{
sind = sqrt(1.0 - pow(cosd, 2.0));
b = pemag * sind;
bm = b * MAU;
pqmag = sqrt(pow(p1mag, 2.0) + pow(pemag, 2.0) - 2.0 * p1mag * pemag * cosd);
zfinl = pemag * cosd;
zinit = -p1mag + zfinl;
xifinl = zfinl / b;
xiinit = zinit / b;
delphi = 2.0 * GS / (bm * c * c) * (xifinl / sqrt(1.0 + pow(xifinl, 2.0)) - xiinit / sqrt(1.0 + pow(xiinit, 2.0)));
delphp = delphi / (1.0 + (pemag / pqmag));
f = delphp * p1mag / sind;
for (j = 0; j < 3; j++)
{
delp = f * (cosd * p1hat[j] + pehat[j]);
pos2[j] = pos[j] + delp;
}
}
return 0;
}
short int aberration(double *pos, double *ve, double lighttime, double *pos2)
{
double p1mag, vemag, beta, dot, cosd, gammai, p, q, r;
if (lighttime == 0.0)
{
p1mag = sqrt(pow(pos[0], 2.0) + pow(pos[1], 2.0) + pow(pos[2], 2.0));
lighttime = p1mag / C;
}
else
p1mag = lighttime * C;
vemag = sqrt(pow(ve[0], 2.0) + pow(ve[1], 2.0) + pow(ve[2], 2.0));
beta = vemag / C;
dot = pos[0] * ve[0] + pos[1] * ve[1] + pos[2] * ve[2];
cosd = dot / (p1mag * vemag);
gammai = sqrt(1.0 - pow(beta, 2.0));
p = beta * cosd;
q = (1.0 + p / (1.0 + gammai)) * lighttime;
r = 1.0 + p;
for (short int j = 0; j < 3; j++)
pos2[j] = (gammai * pos[j] + q * ve[j]) / r;
return 0;
}
short int nutate(double tjd, short int fn, double *pos, double *pos2)
{
double cobm, sobm, cobt, sobt, cpsi, spsi, xx, yx, zx, xy, yy, zy, xz, yz, zz, oblm, oblt, eqeq, psi, eps;
earthtilt(tjd, &oblm, &oblt, &eqeq, &psi, &eps);
cobm = cos(oblm * DEG2RAD);
sobm = sin(oblm * DEG2RAD);
cobt = cos(oblt * DEG2RAD);
sobt = sin(oblt * DEG2RAD);
cpsi = cos(psi / RAD2SEC);
spsi = sin(psi / RAD2SEC);
xx = cpsi;
yx = -spsi * cobm;
zx = -spsi * sobm;
xy = spsi * cobt;
yy = cpsi * cobm * cobt + sobm * sobt;
zy = cpsi * sobm * cobt - cobm * sobt;
xz = spsi * sobt;
yz = cpsi * cobm * sobt - sobm * cobt;
zz = cpsi * sobm * sobt + cobm * cobt;
if (!fn)
{
pos2[0] = xx * pos[0] + yx * pos[1] + zx * pos[2];
pos2[1] = xy * pos[0] + yy * pos[1] + zy * pos[2];
pos2[2] = xz * pos[0] + yz * pos[1] + zz * pos[2];
}
else
{
pos2[0] = xx * pos[0] + xy * pos[1] + xz * pos[2];
pos2[1] = yx * pos[0] + yy * pos[1] + yz * pos[2];
pos2[2] = zx * pos[0] + zy * pos[1] + zz * pos[2];
}
return 0;
}
short int app_star(double tjd, cat_entry *star, double *ra, double *dec)
{
short int error = 0;
double tdb, time2, peb[3], veb[3], pes[3], ves[3], pos1[3], pos2[3],
pos3[3], pos4[3], pos5[3], pos6[3], pos7[3], vel1[3];
if (error = get_earth(tjd, &tdb, peb, veb, pes, ves))
{
*ra = 0.0;
*dec = 0.0;
return error;
}
starvectors(star, pos1, vel1);
proper_motion(T0, pos1, vel1, tdb, pos2);
bary_to_geo(pos2, peb, pos3, &time2);
sun_field(pos3, pes, pos4);
aberration(pos4, veb, time2, pos5);
precession(T0, pos5, tdb, pos6);
nutate(tdb, FN0, pos6, pos7);
vector2radec(pos7, ra, dec);
return 0;
}
void spin(double st, double *pos1, double *pos2)
{
double str, cosst, sinst, xx, yx, xy, yy;
str = st * 15.0 * DEG2RAD;
cosst = cos(str);
sinst = sin(str);
xx = cosst;
yx = -sinst;
xy = sinst;
yy = cosst;
pos2[0] = xx * pos1[0] + yx * pos1[1];
pos2[1] = xy * pos1[0] + yy * pos1[1];
pos2[2] = pos1[2];
return;
}
void pnsw(double gast, double *vece, double *vecs)
{
double dummy, v1[3], v2[3], v3[3];
short int j;
for (j = 0; j < 3; j++)
v1[j] = vece[j];
if (gast == 0.0)
{
for (j = 0; j < 3; j++)
v2[j] = v1[j];
}
else
spin(gast, v1, v2);
for (j = 0; j < 3; j++)
vecs[j] = v2[j];
return;
}
void sidereal_time(double jd_high, double ee, double *gst)
{
double t_hi, t_lo, t, t2, t3, st;
t_hi = (jd_high - T0) / 36525.0;
t_lo = 0;
t = t_hi;
t2 = t * t;
t3 = t2 * t;
st = ee - 6.2e-6 * t3 + 0.093104 * t2 + 67310.54841 + 8640184.812866 * t_lo + 3155760000.0 * t_lo + 8640184.812866 * t_hi + 3155760000.0 * t_hi;
*gst = fmod((st / 3600.0), 24.0);
if (*gst < 0.0)
*gst += 24.0;
return;
}
void equ2hor(double tjd, site_info *location, double ra, double dec, double *zd, double *az, double *rar, double *decr)
{
short int j;
double ujd, dummy, secdiff, tdb, mobl, tobl, ee, dpsi, deps, gast,
sinlat, coslat, sinlon, coslon, sindc, cosdc, sinra, cosra,
uze[3], une[3], uwe[3], uz[3], un[3], uw[3], p[3], pz, pn, pw,
proj, zd0, zd1, refr, cosr, prlen, rlen, pr[3];
ujd = tjd;
tdb2tdt(tjd, &dummy, &secdiff);
tdb = tjd + secdiff / 86400.0;
earthtilt(tdb, &mobl, &tobl, &ee, &dpsi, &deps);
sidereal_time(ujd, ee, &gast);
*rar = ra;
*decr = dec;
sinlat = sin(location->latitude * DEG2RAD);
coslat = cos(location->latitude * DEG2RAD);
sinlon = sin(location->longitude * DEG2RAD);
coslon = cos(location->longitude * DEG2RAD);
sindc = sin(dec * DEG2RAD);
cosdc = cos(dec * DEG2RAD);
sinra = sin(ra * 15.0 * DEG2RAD);
cosra = cos(ra * 15.0 * DEG2RAD);
uze[0] = coslat * coslon;
uze[1] = coslat * sinlon;
uze[2] = sinlat;
une[0] = -sinlat * coslon;
une[1] = -sinlat * sinlon;
une[2] = coslat;
uwe[0] = sinlon;
uwe[1] = -coslon;
uwe[2] = 0.0;
pnsw(gast, uze, uz);
pnsw(gast, une, un);
pnsw(gast, uwe, uw);
p[0] = cosdc * cosra;
p[1] = cosdc * sinra;
p[2] = sindc;
pz = p[0] * uz[0] + p[1] * uz[1] + p[2] * uz[2];
pn = p[0] * un[0] + p[1] * un[1] + p[2] * un[2];
pw = p[0] * uw[0] + p[1] * uw[1] + p[2] * uw[2];
proj = sqrt(pn * pn + pw * pw);
if (proj > 0.0)
*az = -atan2(pw, pn) * RAD2DEG;
if (*az < 0.0)
*az += 360.0;
if (*az >= 360.0)
*az -= 360.0;
*zd = atan2(proj, pz) * RAD2DEG;
return;
}
double julian_date(short int year, short int month, short int day, double hour)
{
long int jd12h = (long)day - 32075L + 1461L * ((long)year + 4800L + ((long)month - 14L) / 12L) / 4L + 367L * ((long)month - 2L - ((long)month - 14L) / 12L * 12L) / 12L - 3L * (((long)year + 4900L + ((long)month - 14L) / 12L) / 100L) / 4L;
double tjd = (double)jd12h - 0.5 + hour / 24.0;
return (tjd);
}
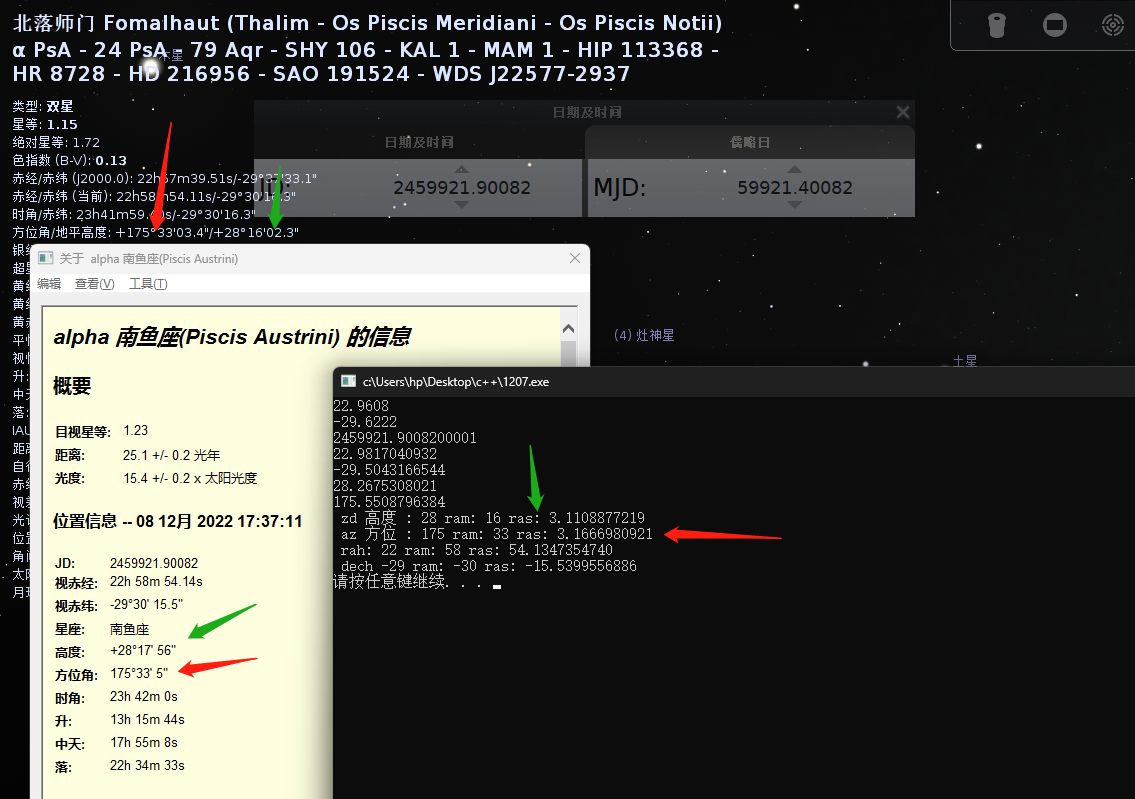
int main()
{
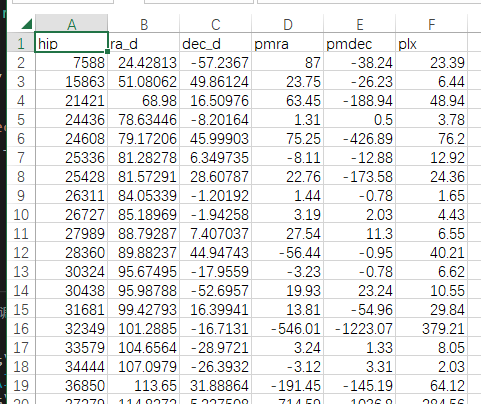
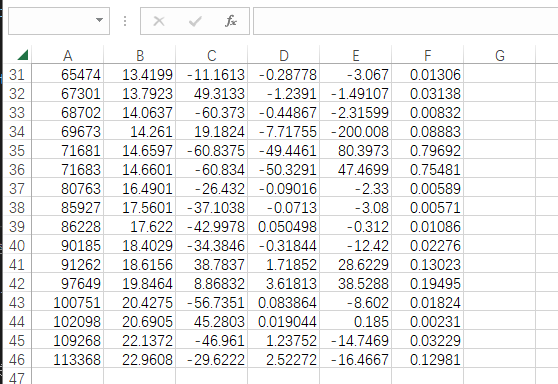
cat_entry hip = {113368, 6.011119421 / TWOPI * 360, -0.516998583 / TWOPI * 360, 328.95, -164.67, 129.81, 0};
cat_entry fk5;
site_info loc = {32.08, 118.8};
transform_hip(&hip, &fk5);
cout << fk5.ra << endl;
cout << fk5.dec << endl;
time_t now;
tm *utc;
double tjd, ra, dec, zd, az, rar, decr, rah;
while (1)
{
now = time(0);
utc = gmtime(&now);
tjd = julian_date(1900 + utc->tm_year, 1 + utc->tm_mon, utc->tm_mday, utc->tm_hour + utc->tm_min / 60.0 + utc->tm_sec / 3600.0);
cout << fixed << setprecision(10) << tjd << endl;
cout << " tjd " << fixed << setprecision(10) << tjd << endl;
app_star(tjd, &fk5, &ra, &dec);
cout << ra << endl
<< dec << endl;
equ2hor(tjd, &loc, ra, dec, &zd, &az, &rar, &decr);
cout << 90 - zd << endl
<< az << endl;
cout << " zd 高度 : " << int(90 - zd) << " ram: " << int((90 - zd - int(90 - zd)) * 60) << " ras: " << 60 * ((90 - zd - int(90 - zd)) * 60 - int((90 - zd - int(90 - zd)) * 60)) << endl;
cout << " az 方位 : " << int(az) << " ram: " << int((az - int(az)) * 60) << " ras: " << 60 * ((az - int(az)) * 60 - int((az - int(az)) * 60)) << endl;
}
rah = ra;
cout << " rah: " << int(rah) << " ram: " << int((rah - int(rah)) * 60) << " ras: " << 60 * ((rah - int(rah)) * 60 - int((rah - int(rah)) * 60)) << endl;
double dech = dec;
cout << " dech: " << (dech < 0 ? "-" : "") << abs(int(dech)) << " ram: " << abs(int((dech - int(dech)) * 60)) << " ras: " << abs(60 * ((dech - int(dech)) * 60 - int((dech - int(dech)) * 60))) << endl;
system("pause");
return 0;
}
|